## deepening
Physical facts can be described from the perspective of different reference systems:**
It is legitimate to describe a physical phenomenon from the point of view of any observer, since ultimately the result of this observation can be transformed into any other reference system. Nevertheless, there are reference systems in which physical phenomena are easier to understand than in others.
Let us take, for example, a so-called inertial system , a system which moves at a constant speed in a predetermined direction; the speed can also be thought of as zero. In this system - let us think of a railway carriage, for example - a passenger will remain seated in his seat without changing his position unless a force acts on him. If, however, the moving train suddenly brakes, the person sitting there may be thrown from his seat without being able to detect any force. He is no longer in an inertial system, but in an accelerated system in which the previously described law of inertia no longer applies. The fact that one can only sit on a chair if one pushes oneself into it, as can be the case in the accelerated system , is not so easy for us to understand.
However, in order to better understand natural processes, the world view has changed again and again throughout history.
At first, people observed from the Earth, which was seen as the center of the world, that the stars all rotated synchronously around the so-called celestial pole, and they were amazed at how precisely their orbits were coordinated with one another. This was easy to understand, however, if they assumed that the stars stood still and only the Earth rotated on its axis.
For many years, people thought about how to explain the observed planetary loops, until Tycho Brahe and Copernicus proposed that the planets orbit the sun instead of the Earth. While Tycho Brahe still saw the Earth as the center of the world, Copernicus placed the sun in its place and had the Earth orbit the sun like the other planets. In both cases, the formation of the planetary loops was self-explanatory.
But why did the celestial bodies float in the middle of space and not fall to the Earth? Newton was able to show that two bodies can remain in a balance of forces despite mass attraction, provided they orbit a common center of gravity at the same angular velocity. In the case of the sun and the earth, this is only about 450 km from the center of the sun due to the much greater mass of the sun. The sun could therefore still be considered approximately as the center of the world. Copernicus’ model thus had an advantage over Tycho Brahe. From the perspective of the heliocentric world view, aberration and the parallax of fixed stars could also be easily explained; both phenomena are based on the annual movement of the earth around the sun.
Eventually it was recognized that the stars themselves were suns, that over 100 billion such suns formed our Milky Way and that they did not stand still, but all moved in this galaxy according to the laws of gravity. At the same time, many other galaxies were discovered in space. But where is the center of the universe? It can hardly be the center of our Milky Way, since it too moves in a galaxy cluster along with other galaxies according to the laws of gravity, similar to how stars move in a galaxy. We now believe that there are many such clusters made up of individual galaxies, which in turn interact with each other gravitationally. We have obviously lost sight of the center of the universe.
Since everything in space seems to be in motion, the only way out was to describe space as an absolute quantity in such a way that its coordinate system had to be thought of as being far removed from all movement.
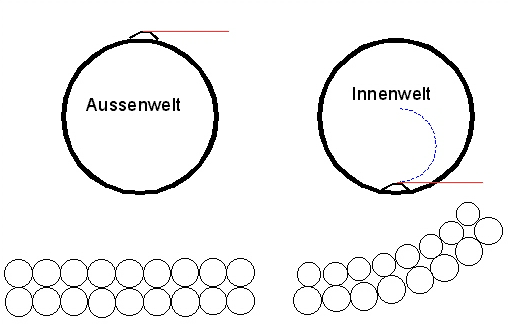
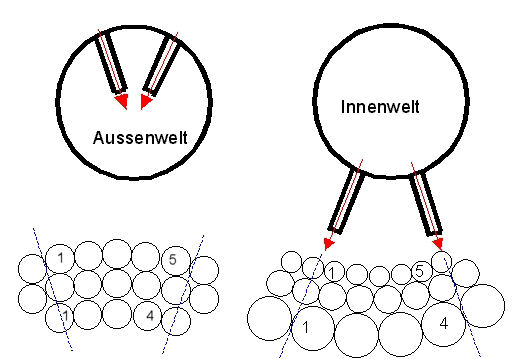
After this digression, let us return to the hollow earth model. As described at the beginning, it reflects the scientific knowledge of the 17th century.**
The geocentric worldview has the earth as a special place at the centre. In the inner world theory, this becomes the shell of the universe. The spatial metric of the hollow earth theory is not limited to the inner area of the globe, but also applies outside, as we have seen in the example of the hole in the earth. Consequently, the earth’s surface is not a necessary prerequisite for this different spatial structure. A transformation that swaps inside with outside can be carried out on any sphere, with the sun as well as with the earth or even on a virtual spherical shell.
The hollow earth model is comparable to Ptolemy’s geocentric worldview. In order to be able to explain the planetary loops in a plausible way, the hollow earth would have to be replaced by a hollow sun, inside of which the planets orbit. Ultimately, it no longer makes sense to define a specific celestial body as the “shell” of the universe. It is sufficient to assume the following spatial structure:
1. There is a distinguished point Omega (we call this area infinity)
2. “Straight lines” run as circular lines through this point
3. Lengths shorten the closer they are to the point Omega.
4. The speed of a force-free body decreases the closer it is to Omega.
These axioms seem unusual and contrived, especially since they do not result in any changes in the description of nature for the observer. They are chosen in such a way that neither the variability of length nor the spatial dependence of speed can ever be experienced. For us, both descriptions are therefore identical.
Why should we even strive for a complicated description of natural processes when there is a simpler way?
Of course, it is obvious that the simpler form of description is preferable here. This description is also considered to be the best form that the human mind has found so far.
On the other hand, we are part of nature and experience it as a component of nature and not from an outside perspective. We simply do not know what nature is like in itself, but only how it presents itself to us. We are not given an absolute view from the outside.
Therefore, it is not impossible that nature may appear simple to us, but in “reality” it could be more complicated. Of course, one could argue that this idea is purely hypothetical and has no effect on our lives. Nevertheless, one thing can be made clear:
Our worldviews are always models of the world; they are time-related, subjective in nature and as uncertain as any human knowledge. Scientific models are therefore not to be seen as reality in itself but as models for